john henry’s hammer: continuous variables III… »
« john henry’s hammer: continuous variables I…
john henry’s hammer: continuous variables II…

Posted on Wednesday 6 January 2016

I got a bit garbled at the end of the last post, so let me start over. I’ve been looking at these two studies, each of which have four groups – placebo and three doses of medication. My table shows three pairwise comparisons in two articles – placebo vs each dose of Brexpiprazole: The results from my simple spreadsheet are nearly identical to those published in the two papers [with one exception discussed later]. But from a statistical point of view, I’ve skipped a step – one that’s often skipped in published RCTs. I have not tested the whole dataset to see if these four grouping are indeed significant [valid]. The null hypothesis is that they aren’t, but rather an arbitrary grouping that doesn’t differ from the overall dataset. The traditional test to verify that there is a statistical basis for this grouping is called a one way anova. If we can’t reject this null hypothesis, the pairwise comparisons are meaningless, no matter how they come out.

BREXPIPRAZOLE Trials in Schizophrenia

STUDY DRUG MEAN SEM σ n p d lower upper
Correll
et al		 placebo -12.01 1.60 21.35 178 – – – –
0.25mg -14.90 2.23 20.80 87 0.3 0.14 -0.120 0.393
2mg -20.73 1.55 20.80 180 <0.0001 0.41 0.204 0.623
4mg -19.65 1.54 20.55 178 0.0006 0.36 0.155 0.574
Kane
et al		 placebo -13.53 1.52 20.39 180 – – – –
1mg -16.90 1.86 20.12 117 0.1588 0.166 -0.067 0.399
2mg -16.61 1.49 19.93 179 0.1488 0.153 -0.054 0.360
4mg -20.00 1.48 19.91 181 0.0022 0.321 0.113 0.529

So how can we do an anova in the absence of the actual data? Again, Internet Calculator to the rescue! Specifically retired Georgetown statistician John C. Pezzullo’s statpages has [among many other wonderful things] this one – Analysis of Variance from Summary Data. This just-what-the-doctor-ordered calculator takes as input the MEAN, either the SD or SEM, and n, and here’s what you end up with [reformatted for clarity]:

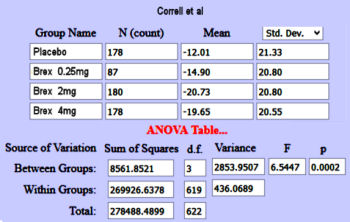
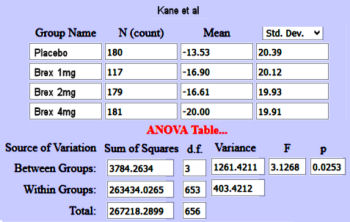
[Note: I checked this with a situation where I had actual data and it was right on the money!] So add this tool to the toolbox [and read his helpful explanation]. Now our table grows a column:

BREXPIPRAZOLE Trials in Schizophrenia

STUDY DRUG MEAN SEM σ n p d lower upper anova p
Correll
et al		 placebo -12.01 1.60 21.35 178 – – – – 0.0002
0.25mg -14.90 2.23 20.80 87 0.3 0.14 -0.120 0.393
2mg -20.73 1.55 20.80 180 <0.0001 0.41 0.204 0.623
4mg -19.65 1.54 20.55 178 0.0006 0.36 0.155 0.574
Kane
et al		 placebo -13.53 1.52 20.39 180 – – – – 0.0025
1mg -16.90 1.86 20.12 117 0.1588 0.166 -0.067 0.399
2mg -16.61 1.49 19.93 179 0.1488 0.153 -0.054 0.360
4mg -20.00 1.48 19.91 181 0.0022 0.321 0.113 0.529

Not bad for data analysis in the absence of data. What’s missing? Well there’s a lot. With packages like SAS, SPSS, R, and data [a big and!], for example, we could do a formal ANCOVA [a general linear model which blends ANOVA and regression] and partition the variance looking at any number of covariates or confounds that might be affecting the outcome. In this case, I’d like to explore the effect of having so many sites. They did that in this article, though their description of the statistical analysis is unnecessarily unintelligible to me. But my little table is enough to be able to make some independent judgements and even compare the results to other studies [see in the land of sometimes[5]…].

Unfortunately, the summary data [MEAN, either the SD or SEM, and n] isn’t always provided in the published articles [though by all rights it should be]. What can we do then? Stay tuned. The Internet hasn’t yet given up all of her secrets!…
  1.  
    James O'Brien, M.D.
    January 6, 2016 | 11:25 AM
     

    Thanks for all of this. We all had biostats at one time but after decades of not using it, it falls into the garbage heap of long term memory like the Krebs Cycle and the imaginary friend Bing Bong in the movie Inside Out.

Sorry, the comment form is closed at this time.